Question

A large population of ALOHA users manages to generate 50 requests/sec, including both originals and retransmissions. Time is slotted in units of 40 msec.

Required:
a. What is the chance of success on the first attempt?
b. What is the probability of exactly k collisions and then a success?
c. What is the expected number of transmission attempts needed?

Answer 1

The answer is below

Step-by-step explanation:

Given that:

Frame transmission time (X) = 40 ms

Requests = 50 requests/sec, Therefore the arrival rate for frame (G) = 50 request * 40 ms = 2 request

a) Probability that there is success on the first attempt =
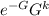`e^(-G)G^k` but k = 0, therefore Probability that there is success on the first attempt =
`e^(-G)=e^(-2)=0.135`

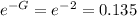
b) probability of exactly k collisions and then a success = P(collisions in k attempts) × P(success in k+1 attempt)

P(collisions in k attempts) = [1-Probability that there is success on the first attempt]^k =
`[1-e^(-G)]^k=[1-0.135]^k=0.865^k`

P(success in k+1 attempt) =
`e^(-G)=e^(-2)=0.135`

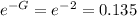
Probability of exactly k collisions and then a success =
`0.865^k0.135`

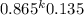
c) Expected number of transmission attempts needed = probability of success in k transmission =
`e^(G)=e^(2)=7.389`