Answer:
(a)
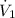 = 0.308 m³/s
= 0.308 m³/s
(b)
 = 0.732 kg/m³
= 0.732 kg/m³
(c) v₂ = 5.94 m/s.
Step-by-step explanation:
(a) The volume flow rate is given by the cross sectional area of the pipe × Velocity of flow of air
Diameter of pipe = 28 cm = 0.28 m
The cross sectional area, A, of the pipe = 0.28²/4×π = 0.0616 m²
Volume flow rate = 5 × 0.0616 = 0.308 m³/s
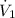 = 0.308 m³/s
= 0.308 m³/s
(b) From the general gas equation, we have;
p₁v₁ = RT₁ which gives;
p₁/ρ₁ = RT₁
ρ₁ = p₁/(RT₁)
Where:
ρ₁ = Density of the air
p₁ = 200 kPa
T₁ = 20 C =
R = 0.287 kPa·m³/(kg·K)
ρ₁ = 200/(0.287 ×293.15) = 2.377 kg/m³
The mass flow rate = Volume flow rate × Density
The mass flow rate,
 = 2.377×0.308 = 0.732 kg/m³
= 2.377×0.308 = 0.732 kg/m³
 = 0.732 kg/m³
= 0.732 kg/m³
(c) The density at exit, ρ₂, is found from the the universal gas equation as follows;
ρ₂ = p₂/(RT₂)
Where:
p₂ = Pressure at exit = 180 kPa
T₂ = Exit temperature = 40°C = 273.15 + 40 = 313.15 K
∴ ρ₂ = 180/(0.287×313.15) = 2.003 kg/m³
 = ρ₂×
= ρ₂×
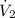
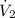 =
=
 /ρ₂ = 0.732/2.003 = 0.366 m³/s
/ρ₂ = 0.732/2.003 = 0.366 m³/s
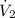 = v₂ × A
= v₂ × A
v₂ =
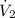 /A = 0.366/0.0616 = 5.94 m/s.
/A = 0.366/0.0616 = 5.94 m/s.
v₂ = 5.94 m/s.