Question

It is believed that the average amount of money spent per U.S. household per week on food is about $98, with standard deviation $11. A random sample of 36 households in a certain affluent community yields a mean weekly food budget of $100. We want to test the hypothesis that the mean weekly food budget for all households in this community is higher than the national average. State the null and alternative hypotheses for this test, the test statistic and determine if the results significant at the 5% level.

Answer 1

a

The null hypothesis is

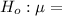`H_o : \mu =`$98

The alternative hypothesis is

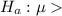`H_a : \mu >`$98

b

test statistics
`t_s = 1.091`

c

The the result of the test statistics is significant

Explanation:

From the question we are told that

The population mean is
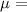`\mu =`$98

The standard deviation is
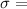`\sigma =`$11

The sample size is
`n = 36`

The sample mean is
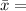`\= x =`$100

The level of significance is
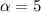`\alpha = 5`% = 0.05

The null hypothesis is

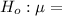`H_o : \mu =`$98

The alternative hypothesis is

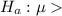`H_a : \mu >`$98

Now the critical values for this level of significance obtained from critical value for z-value table is
`z_\alpha = 1.645`

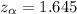
The test statistics is mathematically evaluated as

`t_s = (\= x - \mu)/( (\sigma )/( √(n) ) )`

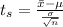
substituting values

`t_s = (100 - 98)/( (11 )/( √(36) ) )`

`t_s = 1.091`

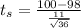
Looking at
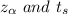`z_\alpha \ and \ t_s` we see that
`z_\alpha \ > t_s` hence the we fail to reject the null hypothesis

hence there is no sufficient evidence to conclude that the mean weekly food budget for all households in this community is higher than the national average.

Thus the the result is significant