Question

What is the volume in cubic inches of the solid figure, rounded to the nearest cubic inch? Do not use units or commas in your answer.

Answer 1

1131 cubic inches.

Explanation:

The front side of the figure contains a rectangle and a semicircle.

Area of rectangle is

`A_1=length* breadth`

`A_1=11* 12`

`A_1=132\text{ in}^2`

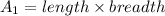
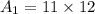
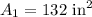
Radius of semicircle is

`r=17-11=6\text{ in}`

Area of semicircle is

`A_2=(1)/(2)\pi r^2`

`A_2=(1)/(2)\pi (6)^2`

`A_2\approx 56.55`

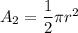
Area of front side is

`A=A_1+A_2=132+56.55=188.55\text{ in}^2`

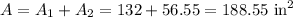
Let front side is the base of prism and height is 6 in. So, volume of given figure is

`V=\text{Base area}* height`

`V=188.55* 6`

`V=1131.3`

`V\approx 1131\text{ in}^3`

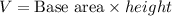
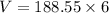
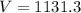
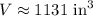
Therefore, the required volume is 1131 cubic inches.