Question

A rod has length 0.900 mm and mass 0.500 kgkg and is pivoted at one end. The rod is not uniform; the center of mass of the rod is not at its center but is 0.500 mm from the pivot. The period of the rod's motion as a pendulum is 1.49 ss. What is the moment of inertia of the rod around the pivot

Answer 1

The moment of inertia is
`I =0.14 \ kg \cdot m^2`

Step-by-step explanation:

From the question we are told that

The length of the rod is
`l = 0.900 \ m`

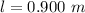
The mass of the rod is
`m = 0.500 \ kg`

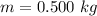
The distance of the center of mass from the pivot is
`d = 0.500 \ m`

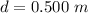
The period of the rod's motion is
`T = 1.49 \ s`

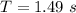
Generally the period of the motion is mathematically represented as

`T = 2 \pi * \sqrt{(I)/(m* g * d) }`

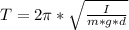
Where
`I` is the moment of inertia about the pivot so making
`I` the subject of formula

`I = [(T)/(2\pi ) ]^2 * m * g * d`

substituting values

`I = [(1.49)/(2* 3.142 ) ]^2 * 0.5 * 9.8 * 0.5`

`I =0.14 \ kg \cdot m^2`