Answer: C) d-6.5 units: M(0.5, 8.5.-2)
Explanation:
Distance between points
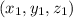 and
and
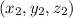 :
:
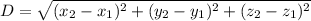
The midpoint of the line joining
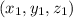 and
and
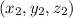 is given by :-
is given by :-
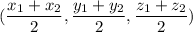
The given points : (3,8, 0) and (-2,9,-4)
Distance between points (3,8, 0) and (-2,9,-4):
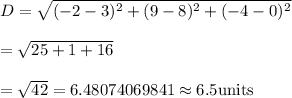
The midpoint of the line joining (3,8, 0) and (-2,9,-4) :

Hence, the correct answer is C) d-6.5 units: M(0.5, 8.5.-2).