Answer:
The percentage is %z
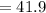 %
%
Explanation:
From the question we are told that
The mean is

The standard deviation is
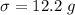
The random number is x = 66 g
Given the the population is normally distributed
The probability is mathematically represented as

Generally the z-score for this population is mathematically represented as
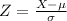
So
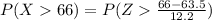
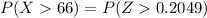
Now the z-value for 0.2049 from the standardized normal distribution table is
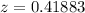
=>
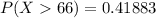
The percentage is
% z

%z
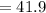 %
%