Answer: a.
![X[a_(n) -v^(n) a_(m) ]](https://img.qammunity.org/2021/formulas/business/college/23hy7e29fxnlfrk3p4h8r3xhwrzaxqf99j.png)
Step-by-step explanation:
The Present Value of the perpetuity for Seth is denoted by;
=
 because Seth receives it for n years.
because Seth receives it for n years.
The Present Value of the perpetuity for Susan is denoted by;
=
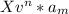 because it is the value after n periods multiplied by the payments received for m periods.
because it is the value after n periods multiplied by the payments received for m periods.
The result is;
=
 -
-
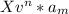
=
![X[a_(n) -v^(n) a_(m) ]](https://img.qammunity.org/2021/formulas/business/college/23hy7e29fxnlfrk3p4h8r3xhwrzaxqf99j.png)