Question:
The volume of a right circular cone with both diameter and height equal to h is 250/7 cm³.
What is the value of h?
Answer:
A. 5
Explanation:
Given
Solid Shape: Cone
Volume = 250/7
Diameter = Height
Required
Find the height of the cone
Provided that the diameter (D) and the height (h) are equal; This implies that
D = h ------ (1)
Also, Diameter (D) = 2 * Radius (r)
D = 2r
Substitute 2r for D in (1)
2r = h
Multiply both sides by ½
½ * 2r = ½ * h
r = ½h
Volume of a cone is calculated by;
Volume = ⅓πr²h
⅓πr²h = 250/7
Substitute ½h for r
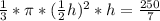
Take π as 22/7, the expression becomes
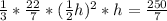
Open the bracket
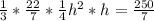
Multiply both sides by 7
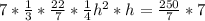

Multiply both sides by 3
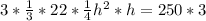
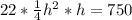
Multiply both sides by 4
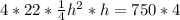

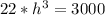
Divide both sides by 22
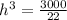
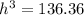
Take cube root of both sides
![h = \sqrt[3]{136.36}](https://img.qammunity.org/2021/formulas/mathematics/high-school/e0e5z2t0jy0p1g7pd32ov6f5m6f7iapj5o.png)

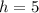 (Approximated)
(Approximated)