Answer:
A 90% confidence interval for the mean weight is [21.78 ounces, 21.98 ounces].
Explanation:
We are given the weights, in the ounces, of a sample of 12 boxes below;
Weights (X): 21.88, 21.76, 22.14, 21.63, 21.81, 22.12, 21.97, 21.57, 21.75, 21.96, 22.20, 21.80.
Firstly, the pivotal quantity for finding the confidence interval for the population mean is given by;
P.Q. =
 ~
~

where,
 = sample mean weight =
= sample mean weight =
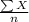 = 21.88 ounces
= 21.88 ounces
s = sample standard deviation =
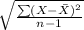 = 0.201 ounces
= 0.201 ounces
n = sample of boxes = 12
 = population mean weight
= population mean weight
Here for constructing a 90% confidence interval we have used a One-sample t-test statistics because we don't know about population standard deviation.
So, 90% confidence interval for the population mean,
 is ;
is ;
P(-1.796 <
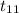 < 1.796) = 0.90 {As the critical value of t at 11 degrees of
< 1.796) = 0.90 {As the critical value of t at 11 degrees of
freedom are -1.796 & 1.796 with P = 5%}
P(-1.796 <
 < 1.796) = 0.90
< 1.796) = 0.90
P(
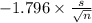 <
<
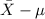 <
<
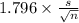 ) = 0.90
) = 0.90
P(
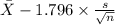 <
<
 <
<
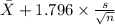 ) = 0.90
) = 0.90
90% confidence interval for
 = [
= [
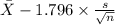 ,
,
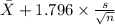 ]
]
= [
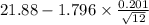 ,
,
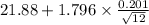 ]
]
= [21.78, 21.98]
Therefore, a 90% confidence interval for the mean weight is [21.78 ounces, 21.98 ounces].