Answer:
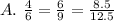
Explanation:
Given
Let the two triangles be A and B
Sides of A: 4, 6 and 8.5
Sides of B: 6, 9 and 12.5
Required
Which set of ratio determines the dilation
To determine the dilation of a triangle over another;
We simply divide the side of a triangle by a similar side on the other triangle;
From the given parameters,
A ------------------B
4 is similar to 6
6 is similar to 9
8.5 is similar to 12.5
Ratio of dilation is as follows;
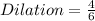
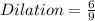
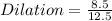
Combining the above ratios;
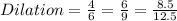
From the list of given options, the correct option is A,