Question

8. rajis annual salary ranges from $25 325 in the 1st year to $34 445 in the 7th year. The salaries in this range form an arithmetic sequence? a) Determine the raise the person can expect each year. b) What is the total amount the person will earn in the 7 years?

Answer 1

a) $1520

b)
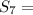`S_7 =` $209195

Explanation:

The range of salaries forms an arithmetic sequence with the first term as 25325 and its 7th term as 34445:

a) The raise the person can get each year is the common difference of the progression, d.

The nth term of an arithmetic progression is given generally as:

`a_n = a + (n - 1)d`

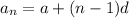
where a = first term = 25325

d = common difference

Therefore, the 7th term (34445) will be:

34445 = 25325 + (7 - 1)d

34445 = 25325 + 6d

=> 6d = 34445 - 25325 = 9120

d = 9120 / 6 = $1520

Therefore, the raise the person gets each year (common difference) is $1520.

b) The total amount the person will earn after 7 years is the sum of the salaries of all 7 yeas.

The sum of an arithmetic progression up to the nth term is given as:

`S_n = (n)/(2)(2a + (n - 1)d)\\ \\`

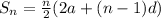
Therefore, the sum of the person's salary for the 7 years is:

`S_7 = (7)/(2)(2 * 25325 + (7 - 1)1520)\\ \\S_7 = 3.5 (50650 + 6(1520))\\\\S_7 = 3.5 (50650 + 9120)\\\\S_7 = 3.5 * 59770\\`

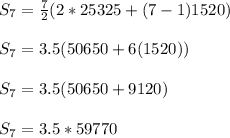
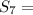`S_7 =` $209195

That is the total amount of salary after 7 years