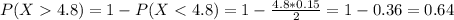Question

Consider the density curve plotted below:

Find PX < 6.4):
Find P(X> 4.8):

Answer 1

`P(X<6.4)= (6.4*0.2)/(2)= 0.64`

`P(X>4.8) =1-P(X<4.8)= 1- (4.8*0.15)/(2)= 1-0.36= 0.64`

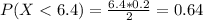
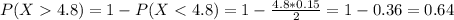
Explanation:

Part a

We want to find:

`P(X<6.4)`

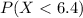
And we just need to find the area below the curve until x=6.4, since we have a triangle we can do this:

`P(X<6.4)= (6.4*0.2)/(2)= 0.64`

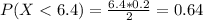
Part b

For this case we want to find this probability:

`P(X>4.8)`

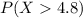
And we can use the complement rule and we got:

`P(X>4.8) =1-P(X<4.8)= 1- (4.8*0.15)/(2)= 1-0.36= 0.64`