Answer:
a) CD = 41 m
b) 77.32°
c) 1380 square metres
Explanation:
We can divide the trapezium as shown in the diagram below.
a) To find CD, we use Pythagoras Rule:

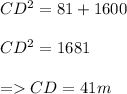
b) To find <BCD, we use trigonometric function SOHCAHTOA:
sin(BCD) = opp / hyp
sin(BCD) = 40 / 41
sin(BCD) = 0.9756
=> <BCD = 77.32°
c) The area of a trapezium is given as:
A = 1/2 (a + b) * h
where h = height = 40 m
a = top length = 30 m
b = bottom length = 39 m
A = 1/2 * (30 + 39) * 40
A = 1/2 * 69 * 40
A = 1380 square metres