Answer:
For this problem we have the following function given:

In order to clasify the function as linear or quadratic we need to see the higher exponent for x in the equation and for this case we see that the higher exponent is 2 since we have in the first term
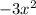 . So then we can classify this equation as quadratic
. So then we can classify this equation as quadratic
Quadratic term: -3
Linear term: -29
Constant term: 30
Explanation:
For this problem we have the following function given:

In order to clasify the function as linear or quadratic we need to see the higher exponent for x in the equation and for this case we see that the higher exponent is 2 since we have in the first term
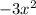 . So then we can classify this equation as quadratic
. So then we can classify this equation as quadratic
Quadratic term: -3
Linear term: -29
Constant term: 30