Question

A steam power plant operates on the reheat Rankine cycle. Steam enters the highpressure turbine at 12.5 MPa and 550°C at a rate of 7.7 kg/s and leaves at 2 MPa. Steam is then reheated at constant pressure to 450 °C before it expands in the low-pressure turbine. The isentropic efficiencies of the turbine and the pump are 85 percent and 90 percent, respectively. Steam leaves the condenser as a saturated liquid. If the moisture content of the steam at the exit of the turbine is not to exceed 5 percent, determine (a) the condenser pressure, (b) the net power output, and (c) the thermal efficiency

Answer 1

A) condenser pressure = 9.73 kPa,

B) 10242 kw

C) 36.9%

Step-by-step explanation:

given data

entrance pressure of steam = 12.5 MPa

temperature of steam = 550⁰c

flow rate of steam = 7.7 kg/s

outer pressure = 2 MPa

reheated steam temperature = 450⁰c

isentropic efficiency of turbine( nt ) = 85% = 0.85

isentropic efficiency of pump = 90% = 0.90

From steam tables

at 12.5 MPa and 550⁰c ; h3 = 3476.5 kJ/kg, S3 = 6.6317 kJ/kgK

also for an Isentropic expansion

S4s = S3 .

therefore when S4s = 6.6317 kJ/kg and P4 = 2 MPa

h4s = 2948.1 kJ/kg

nt = 0.85

nt (0.85) =
`(h3-h4)/(h3-h4s)` =
`(3476.5 - h4)/(3476.5 - 2948.1)`

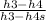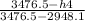
making h4 subject of the equation

h4 = 3476.5 - 0.85 (3476.5 - 2948.1)

h4 = 3027.3 kj/kg

at P5 = 2 MPa and T5 = 450⁰c

h5 = 3358.2 kj/kg, s5 = 7.2815 kj/kgk

at P6 , x6 = 0.95 and s5 = s6

using nt = 0.85 we can calculate for h6 and h6s

from the chart attached below we can see that

p6 = 9.73 kPa, h6 = 2463.3 kj/kg

B) the net power output

solution is attached below

c) thermal efficiency

thermal efficiency = 1 -
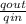`(qout)/(qin)` = 1 - ( 2273.7/ 3603.8) = 36.9% ≈ 37%