Question

A walking path across a park is represented by the equation A walking path across a park is represented by the equation y= -3x-3. A New path will be built perpendicular to this path. The Paths will intersect at a point paths will intersect at a point (-3, 6). Identify The equation that represents the new path.

Answer 1

The equation representing the new path is;

`y = (1)/(3) \cdot x + 7`

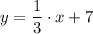
Explanation:

The equation of the first walking park across the park is y = -3·x - 3

By comparison to the equation of a straight line, y = m·x + c, where m = the slope of the line, the slope of the line y = -3·x - 3 is -3

The park's new walking path direction = Perpendicular to first walking path

A line perpendicular to a line of (as example) y = m₁·x + c has a slope of -1/m

∴ The park's new walking path slope = -1/(Slope of first path) = -1/(-3) = 1/3

The point the paths will intersect = (-3, 6)

The equation of the line is found by recalling that
`Slope, \, m_1 =(y_(2)-y_(1))/(x_(2)-x_(1))`

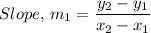
Where:

y₂ and x₂ are coordinates of a point on the new walking path

y₁ and x₁ are coordinates of a point on the new walking path intersecting the first walking path

Given that (-3, 6) is the intersection of the two walking paths, therefore, it is a point on the new walking path and we can say x₁ = -3, y₁ = 6

Therefore, we have;

`Slope, \, m_1 =(y_(2)-6)/(x_(2)-(-3)) = (y_(2)-6)/(x_(2)+3) =(1)/(3)`

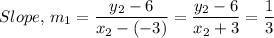
Which gives;

(y₂ - 6) × 3 = x₂ + 3

y₂ - 6 = (x₂ + 3)/3

y₂ = (x₂ + 3)/3 + 6 = 1/3·x₂ + 1 + 6 = 1/3·x₂ + 7

Which gives the equation representing the new path as
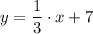`y = (1)/(3) \cdot x + 7`.