Question

(a) A duct for an air conditioning system has a rectangular cross section of 1.8 ft × 8 in. The duct is fabricated from galvanized iron. Determine the Reynolds number for a flow rate of air of 5400 cfm at 100 °F and atmospheric pressure (g=0.0709 lbf/ft3 u=1.8×10-4ft2/s and m=3.96×10-7lbf.s/ft2) (9 points)

Answer 1

Reynolds number = 654350.92

Step-by-step explanation:

Given data:

Cross section of rectangular cross section = 1.8ft * 8 in ( 8 in = 2/3 ft )

Flow rate of air = 5400 cfm = 90 ft^3 / sec

v ( kinematic viscosity of air ) = 1.8*10^-4 ft^2/s

Reynolds number

Re = VDn / v

Dn ( hydraulic diameter ) = 4A / P

where A = area, P = perimeter

a = 1.8 ft ( length )

b = 2/3 ft ( width )

hence Dn =
`(4(ab))/(2(a+b))` =
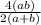`(4(1.8*0.6667)/(2(1.8+0.6667))` = 0.9729 ft

V ( velocity of air flow ) =
`(Q)/(\pi /4 * Dn^2 )` =
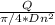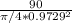`(90)/(\pi /4 * 0.9729^2 )` = 121.064 ft/sec

back to Reynolds equation

Re = VDn / v -------------- equation 1

V = 121.064 ft/sec

Dn = 0.9729 ft

v = 1.8*10^-4 ft^2/s

insert the given values into equation 1

Re = (121.064 * 0.9729 ) / 1.8*10^-4

= 654350.92