Question

A block is attached to a horizontal spring and it slides back and forth in simple harmonic motion on a frictionless horizontal surface. At one extreme end of the oscillation cycle, where the block comes to a momentary halt before reversing the direction of its motion, another block is placed on top of the first block without changing its zero velocity. The simple harmonic motion then continues. What happens to the amplitude and the angular frequency of the ensuing motion of the two-block system

Answer 1

A = A₀ , w = w₀/√2

Step-by-step explanation:

This is a problem that we must solve with Newton's second law. They indicate that at the end of the initial movement where the speed is zero, add a mass to the block, we assume that it has the same mass, therefore the total mass is m_total = 2 m. Let's write Newton's second law at this point

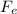`F_(e)` = m_total a

the elastic force is

F_{e} = - k x

acceleration is

a = d²x / dt²

we substitute

- k x = m_total d²x / dt²

d²x / dt² + (k / m_total) x = 0

we substitute

d²x / dt² + (k /2m) x = 0

the solution to this differential equation is

x = A cos (wt + Ф)

where

w = √ (k / 2m)

to find the constant Ф we use the velocity

v = dx / dt = - Aw sin (wt + Ф)

At the most extreme point and when the new movement begins (t = 0) they indicate that v = 0

0 = - A w sin Ф

for this expression to be zero the sine must be zero therefore Ф = 0

when replacing

x = A cos (wt)

w = 1 /√2 √ (k / m)

if we want to relate to the initial movement (before placing the block)

w₀ = √ (k / m)

w = w₀ /√ 2

The amplitude of the movement is the distance from the equilibrium point to where the movement begins, in this case it is the same as in the initial movement

A = A₀

the subscript is used to refer to the oscillations before placing the second block

we substitute to have the final equation

x = A₀ cos (w₀ t /√2)

A = A₀

w = w₀/√2