Question

Suppose you titrate 25.00 mL of 0.200 M KOBr with 0.200M H2SO4. The pH at half-equivalence point is 7.75 a). What is the initial pH of the 25.00mL of 0.200M KOBr mentioned above

Answer 1

Approximately
`10.88`.

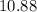Step-by-step explanation:

Equilibrium constant

`\rm OBr^(-)` can act as a weak Bronsted-Lowry base:

`\rm OBr^(-)\; (aq) + H_2O\; (l) \rightleftharpoons HOBr\; (l) + OH^(-)\; (aq)`.

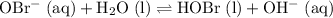(Side note: the state symbol of
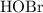`\rm HOBr` in this equation is
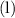`\rm (l)` (meaning liquid) because
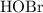`\rm HOBr` is a weak acid.)

However, the equilibrium constant of this reaction,
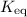`K_\text{eq}`, isn't directly given. The idea is to find
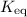`K_\text{eq}` using the
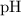`\rm pH` value at the half-equivalence point. Keep in mind that this system is at equilibrium all the time during the titration. If temperature stays the same, then the same
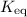`K_\text{eq}` value could also be used to find the
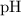`\rm pH` of the solution before the acid was added.

At equilibrium:

`\displaystyle K_\text{eq} = ([\rm HOBr\; (l)]\cdot [\rm OH^(-)\; (aq)])/([\rm OBr^(-)\; (aq)])`.

At the half-equivalence point of this titration, exactly half of the base,
`\rm OBr^(-)`, has been converted to its conjugate acid,
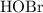`\rm HOBr`. Therefore, the half-equivalence concentration of
`\rm OBr^(-)` and
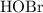`\rm HOBr` should both be equal to one-half the initial concentration of
`\rm OBr^(-)`.

As a result, the half-equivalence concentration of
`\rm OBr^(-)` and
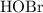`\rm HOBr` should be the same. The expression for
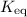`K_\text{eq}` can thus be simplified:

`\begin{aligned}& K_\text{eq} \\&= \frac{\left(\text{half-equivalence $[\rm HOBr\; (l)]$}\right)\cdot \left(\text{half-equivalence $[\rm OH^(-)\; (aq)]$}\right)}{\text{half-equivalence $[\rm OBr^(-)\; (l)]$}}\\ &=\text{half-equivalence $[\rm OH^(-)\; (aq)]$}\end{aligned}`.

In other words, the
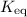`K_\text{eq}` of this system is equal to the
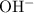`\rm OH^(-)` concentration at the half-equivalence point. Assume that
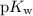`\rm p\mathnormal{K}_\text{w}` the self-ionization constant of water, is
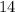`14`. The concentration of
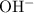`\rm OH^(-)` can be found from the
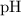`\rm pH` value:

`\begin{aligned}& \text{half-equivalence $[\rm OH^(-)\; (aq)]$} \\ &= 10^{\rm pH - p\mathnormal{K}_\text{w}}\;\rm mol \cdot L^(-1) \\ &= 10^(7.75 - 14)\; \rm mol \cdot L^(-1)\\ &= 10^(-6.25)\; \rm mol \cdot L^(-1)\end{aligned}`.

Therefore,
`\begin{aligned} K_\text{eq} &= 10^(-6.25)\end{aligned}`.

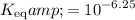Initial pH of the solution

Again, since
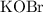`\rm KOBr` is a soluble salt, all that
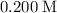`0.200\; \rm M` of
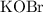`\rm KOBr` in this solution will be in the form of
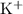`\rm K^(+)` and
`\rm OBr^(-)` ions. Before any hydrolysis takes place, the concentration of
`\rm OBr^(-)` should be equal to that of
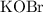`\rm KOBr`. Therefore:

`\text{$[\rm OBr^(-)\; (aq)]$ before hydrolysis} = 0.200\; \rm M`.

Let the equilibrium concentration of
`[\rm OH^(-)\; (aq)]` be
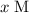`x\; \rm M`. Create a RICE table for this reversible reaction:

.

Assume that external factors (such as temperature) stays the same. The
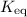`K_\text{eq}` found at the half-equivalence point should apply here, as well.

`\displaystyle K_\text{eq} = ([\rm HOBr\; (l)]\cdot [\rm OH^(-)\; (aq)])/([\rm OBr^(-)\; (aq)])`.

At equilibrium:

`\displaystyle ([\rm HOBr\; (l)]\cdot [\rm OH^(-)\; (aq)])/([\rm OBr^(-)\; (aq)]) = (x^2)/(0.200 + x)`.

Assume that
`x` is much smaller than
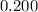`0.200`, such that the denominator is approximately the same as
`0.200`:

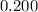`\displaystyle ([\rm HOBr\; (l)]\cdot [\rm OH^(-)\; (aq)])/([\rm OBr^(-)\; (aq)]) = (x^2)/(0.200 + x) \approx (x^2)/(0.200)`.

That should be equal to the equilibrium constant,
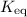`K_\text{eq}`. In other words:

`\displaystyle (x^2)/(0.200) \approx K_\text{eq} = 10^(-6.25)`.

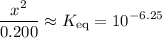Solve for
`x`:

`x \approx 3.35* 10^(-4)`.

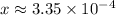In other words, the
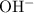`\rm OH^(-)` before acid was added was approximately
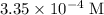`3.35* 10^(-4)\; \rm M`, which is the same as
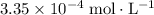`3.35* 10^(-4)\; \rm mol \cdot L^(-1)`. Again, assume that
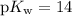`\rm p\mathnormal{K}_\text{w} = 14`. Calculate the
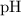`\rm pH` of that solution:

`\begin{aligned}\rm pH &= \rm p\mathnormal{K}_\text{w} + \log [\mathrm{OH^(-)}] \approx 10.88\end{aligned}`.

(Rounded to two decimal places.)