Question

A 32-cm-long solenoid, 1.8 cm in diameter, is to produce a 0.30-T magnetic field at its center. If the maximum current is 4.5 A, how many turns must the solenoid have?

Answer 1

16,931 turns

Step-by-step explanation:

The magnetic field produced is expressed using the formula

`B = (\mu_0NI)/(L)`

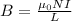
B is the magnetic field = 0.30T

I is the current produced in the coil = 4.5A

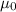`\mu_0` is the magnetic permittivity in vacuum = 1.26*10^-6Tm/A

L is the length of the solenoid = 32 cm = 0.32 m

N is the number of turns in the solenoid.

Making N the subject of the formula from the equation above;

`B = (\mu_0NI)/(L)\\\\BL = \mu_0NI\\\\Dividing\ both\ sides \ by \ \mu_0I\\\\(BL)/(\mu_0I) =(\mu_oNI)/(\mu_0I) \\\\`

`N = (BL)/(\mu_0I)`

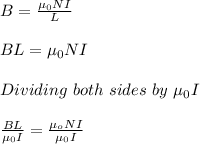
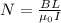
Substituting the give values to get N;

`N = (0.3*0.32)/(1.26*10^(-6) * 4.5)\\\\N = (0.096)/(0.00000567) \\\\N = 16,931.21`

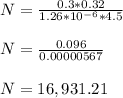
The number of turns the solenoid must have is approximately 16,931 turns