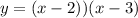Answer:
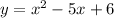
And for this case we want to find the zeros or x interceps r and s so we want to rewrite the function on this way:
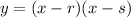
The reason why we have two zeros is because the degree of the polynomial is 2. If we find two numbers that adding we got -5 and multiplied 6 we solve the problem. For this case the solution is r =3, s =2
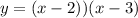
Explanation:
For this problem we have the following polynomial given:
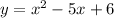
And for this case we want to find the zeros or x interceps r and s so we want to rewrite the function on this way:
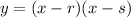
The reason why we have two zeros is because the degree of the polynomial is 2. If we find two numbers that adding we got -5 and multiplied 6 we solve the problem. For this case the solution is r =3, s =2