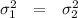Answer:
Explanation:
Given that:
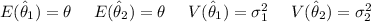
If we are to consider the estimator

a. Then, for
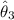 to be an unbiased estimator ; Then:
to be an unbiased estimator ; Then:


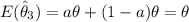
b) If
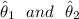 are independent
are independent
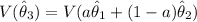
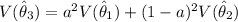
Thus; in order to minimize the variance of
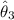 ; then constant a can be determined as :
; then constant a can be determined as :
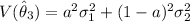
Using differentiation:
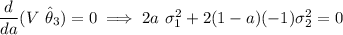
⇒
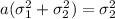
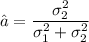
This implies that
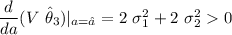
So,
 is minimum when
is minimum when
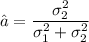
As such;
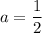 if
if