Question

Suppose ACT Reading scores are normally distributed with a mean of 21 and a standard deviation of 6.2. A university plans to admit students whose scores are in the top 30%. What is the minimum score required for admission

Answer 1

24.25

Explanation:

The minimum admission score is at the 70th percentile of the normal distribution which, according to a z-score table, corresponds to a z-score of 0.524.

The z-score, for any given value X, is determined by:

`z=(X-\mu)/(\sigma)`

If the mean score is 21 and the standard distribution is 6.2, the minimum required score for admission is:

`0.524=(X-21)/(6.2)\\X=24.25`

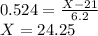
The minimum score required for admission is 24.25.