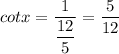Answer:

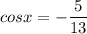
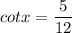
Explanation:
Given that:
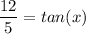

i.e. x is in 3rd quadrant. So tan is positive.
To find:
sin(x), cos(x), and cot(x).
Solution:
Given that:
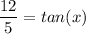
We know by trigonometric identities that:
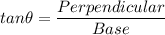
Comparing with the given values:
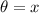
Perpendicular = 12 units
Base = 5 units
Using pythagorean theorem, we can find out hypotenuse:
According to pythagorean theorem:
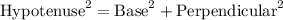

We can easily find out the values of:
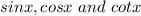
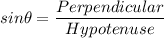
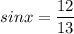
Given that x is in 3rd quadrant, sinx will be negative.
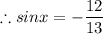
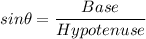
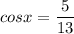
Given that x is in 3rd quadrant, cosx will be negative.
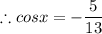
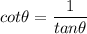
Given that x is in 3rd quadrant, cotx will be positive.