Answer:
For this case we have this function:

We can factor the denominator and we got:
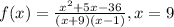
And since we can't divide by 0 then the value of x can't be 1 or -9 so then the best answer for this case would be:
Continuous at every real number except x=1 and x=-9
Explanation:
For this case we have this function:

We can factor the denominator and we got:
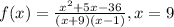
And since we can't divide by 0 then the value of x can't be 1 or -9 so then the best answer for this case would be:
Continuous at every real number except x=1 and x=-9