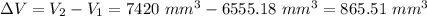Question

Regulation baseballs have a diameter that is either 23.2 mm or 24.2 mm. What is the difference in volume of the baseballs? Round to the nearest hundredth. Use pi equals 3.14. V equals ____________ mm cubed Type your numerical answer (without units) below.

Answer 1

ΔV = 865.51 mm^3

Explanation:

In order to calculate the difference in volume between both baseballs you use the following formula for the volume of a sphere:

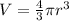`V=(4)/(3)\pi r^3` (1)

where r is the radius of he sphere.

You calculate the volume of each sphere:

First baseball:

radius = 23.2mm/2 = 11.61mm

`V_1=(4)/(3)\pi (11.61mm)^3=6555.18\ mm^3`

Second baseball:

radius = 24.2mm/2 = 12.1mm

`V_2=(4)/(3)\pi (12.10)^3=7420.70\ mm^3`

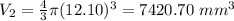
Then, the difference in the volumen of both spheres is:

`\Delta V=V_2-V_1=7420\ mm^3-6555.18\ mm^3=865.51\ mm^3`