Question

Find the distance between the points by filling in the steps. The first one is done for you.

​

Answer 1

Q.2
`4\sqrt2` units

Q.3
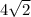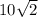`10\sqrt2` units

Explanation:

Given that points:

C(-1, 3) and D (-5, 7)

E(0, -5) and F(10, -15)

To find:

Q.2. Distance between C and D

Q.3. Distance between E and F

Solution:

First of all, let us solve the question marked as number 2 in the attached image.

C(-1, 3) and D (-5, 7)

`x_2 = -5\\x_1 = -1\\y_2 = 7\\y_1 = 3`

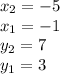
To find the distance 'd' between the points, we can use distance formula:

`d = √((x_2-x_1)^2+(y_2-y_1)^2)`

`d = √((-5-(-1))^2+(7-3)^2)\\\Rightarrow d = √((-4)^2+(4)^2)\\\Rightarrow d =√(16+16)\\\Rightarrow d =√(32) = 4\sqrt2\ units`

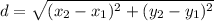
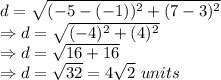
First of all, let us solve the question marked as number 3 in the attached image.

E(0, -5) and F(10, -15)

`x_2 = 10\\x_1 = 0\\y_2 = -15\\y_1 = -5`

To find the distance 'd' between the points, we can use distance formula:

`d = √((x_2-x_1)^2+(y_2-y_1)^2)`

`d = √((10-0)^2+(-15-(-5))^2)\\\Rightarrow d = √(10^2+(-10)^2)\\\Rightarrow d =√(100+100)\\\Rightarrow d =√(200) = 10\sqrt2\ units`

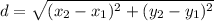
Please find attached image for the answers filled in the boxes.