Question

If the current in the circuit decreases, what does that mean about the rate at which the charge(and voltage) change in a capacitor?

2. the exponent of the exponential function contains RC for the given circuit. who's is a constant. use units R and C to find units of RC. write ohms in terms of volts and amps and write farads in terms of volts and coulombs. Simplify
units of RC are__________

Answer 1

`1. charge Q, on the capacitor increases, while the current will decrease

2. τ = t = secs

Step-by-step explanation:

1. consider RC of a circuit to be am external source

voltage across the circuit is given as

v =v₀(1 -
`e^{(t)/(τ) }`)

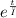where v = voltage

v₀ = peak voltage

t = time taken

τ= time constant

as the charge across the capacitor increases, current decreases

the charge across the circuit is given as

Q= Q₀(1 -
`e^{(t)/(τ) }`)

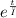charge Q is inversely proportional to the current I

hence the charge across the circuit increases

2. τ = RC

unit of time constant, τ,

= Ω × F

=
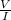`(V)/(I)` ˣ
`(C)/(V)`

=
`(C)/(A)`

=
`(C)/(C/t)`

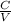
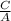
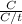
τ = t = secs