Question

Ask Your Teacher An electric utility company supplies a customer's house from the main power lines (120 V) with two copper wires, each of which is 34.0 m long and has a resistance of 0.109 Ω per 300 m. (a) Find the potential difference at the customer's house for a load current of 116 A.

Answer 1

The potential difference at the customer's house is 117.1 V.

Step-by-step explanation:

a) The potential difference at the customer's house can be calculated as follows:

`\Delta V_(h) = \Delta V_(p) - \Delta V_(l)`

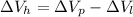
Where:

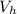`V_(h)`: is the potential difference at the customer's house

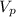`V_(p)`: is the potential difference from the main power lines = 120 V

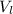`V_(l)`: is the potential difference from the lines

`\Delta V_(h) = \Delta V_(p) - IR`

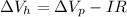
The resistance, R, is:

`(0.109 \Omega)/(300 m)*2*34.0 m = 0.025 \Omega`

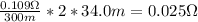
Now, the potential difference at the customer's house is:

`\Delta V_(h) = 120 V - 116A*0.025 \Omega = 117.1 V`

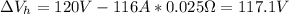
Therefore, the potential difference at the customer's house is 117.1 V.

I hope it helps you!