Question

The rotor of a gas turbine is rotating at a speed of 7000 rpm when the turbine is shut down. It is observed that 3.5 minutes is required for the rotor to coast to rest. Assuming uniformly accelerated motion, determine the number of revolutions that the rotor executes before coming to rest. Hint: there will be a large number of rotations.

Answer 1

The rotor of the gas turbine rotates 12250 revolutions before coming to rest.

Step-by-step explanation:

Given that rotor of gas turbine is decelerating at constant rate, it is required to obtained the value of angular acceleration as a function of time, as well as initial and final angular speeds. That is:

`\dot n = \dot n_(o) + \ddot n \cdot t`

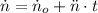
Where:

`\dot n_(o)` - Initial angular speed, measured in revolutions per minute.

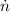`\dot n` - Final angular speed, measured in revolutions per minute.

`t` - Time, measured in minutes.

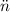`\ddot n` - Angular acceleration, measured in revoiutions per square minute.

The angular acceleration is now cleared:

`\ddot n = (\dot n - \dot n_(o))/(t)`

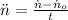
If
`\dot n_(o) = 7000\,(rev)/(min)`,
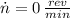`\dot n = 0\,(rev)/(min)` and
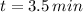`t = 3.5\,min`, the angular acceleration is:

`\ddot n = (0\,(rev)/(min)-7000\,(rev)/(min) )/(3.5\,min)`

`\ddot n = -2000\,(rev)/(min^(2))`

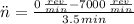
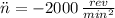
Now, the final angular speed as a function of initial angular speed, angular acceleration and the change in angular position is represented by this kinematic equation:

`\dot n^(2) = \dot n_(o)^(2) + 2\cdot \ddot n \cdot (n-n_(o))`

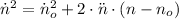
Where
`n` and
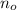`n_(o)` are the initial and final angular position, respectively.

The change in angular position is cleared herein:

`n-n_(o) = (\dot n^(2)-\dot n_(o)^(2))/(2\cdot \ddot n)`

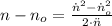
If
`\dot n_(o) = 7000\,(rev)/(min)`,
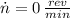`\dot n = 0\,(rev)/(min)` and
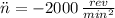`\ddot n = -2000\,(rev)/(min^(2))`, the change in angular position is:

`n-n_(o) = (\left(0\,(rev)/(min) \right)^(2)-\left(7000\,(rev)/(min) \right)^(2))/(2\cdot \left(-2000\,(rev)/(min^(2)) \right))`

`n-n_(o) = 12250\,rev`

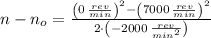
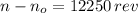
The rotor of the gas turbine rotates 12250 revolutions before coming to rest.