Answer:
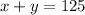 (1)
(1)
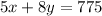 (2)
(2)
We can solve for y from equation (1) and we got:
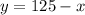 (3)
(3)
And replacing (3) into (2) we got:

And solving for x we got:
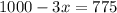
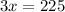
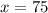
And solving for y from (3) we got:

And the solution would be x = 50 and y =75
Explanation:
For this problem we have the following system of equations:
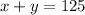 (1)
(1)
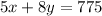 (2)
(2)
We can solve for y from equation (1) and we got:
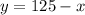 (3)
(3)
And replacing (3) into (2) we got:

And solving for x we got:
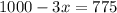
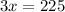
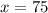
And solving for y from (3) we got:

And the solution would be x = 50 and y =75