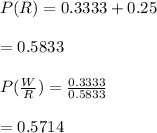Question

urn I contains 1 red chip and 2 white chips; urn II contains 2 red chipsand 1 white chip. One chip is drawn at random from urn I and transferred to urnII. Then one chip is drawn from urn II. Suppose that a red chip is selected from urnII. What is the probability that the chip transferred was white

Answer 1

Following are the answer to this question:

Explanation:

In the question first calls the W if the transmitted chip was white so, the W' transmitted the chip is red or R if the red chip is picked by the urn II.

whenever a red chip is chosen from urn II, then the probability to transmitters the chip in white is:

`P((w)/(R)) = (P(W\cap R))/(P(R)) \ \ \ \ \ _(Where)\\\\P(R) = P(W\cap R) + P(W'\cap R) \\`

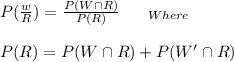
The probability that only the transmitted chip is white is therefore
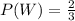`P(W) = (2)/(3)\\`, since urn, I comprise 3 chips and 2 chips are white.

But if the chip is white so, it is possible that urn II has 4 chips and 2 of them will be red since urn II and 2 are now visible, and it is possible to be:
`P((R)/(W)) = (2)/(3)`

`P(W\cap R) = P(W) * P((R)/(W)) \\`

`= (2)/(3)* (2)/(4) \\\\= (2)/(3)* (1)/(2) \\\\= (2)/(3)* (1)/(1) \\\\=(1)/(3)\\\\= 0.333`

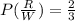
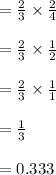
Likewise, the chip transmitted is presumably red
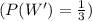`(P(W')= (1)/(3))`and the chip transferred is a red chip of urn II
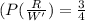`(P((R)/(W'))= (3)/(4)`, and a red chip is likely to be red
`((R)/(W'))`.

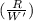Finally,
`P(W'\cap R) = P(W') * P((R)/(W'))\\`

`= (1)/(3) * (3)/(4) \\\\ = (1)/(1) * (1)/(4) \\\\=(1)/(4)\\\\= 0.25`

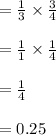
The estimation of
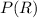`P(R)` and
`P((W )/(R))` as:

`P(R) = 0.3333 + 0.25\\\\ \ \ \ \ \ \ \ \ \ = 0.5833 \\\\ P((W)/(R)) = (0.3333)/(0.5833) \\\\\ \ \ \ \ \ \ = 0.5714`