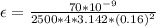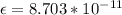Answer:
The permittivity of rubber is
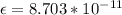
Step-by-step explanation:
From the question we are told that
The magnitude of the point charge is
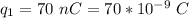
The diameter of the rubber shell is
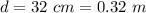
The Electric field inside the rubber shell is
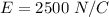
The radius of the rubber is mathematically evaluated as
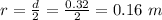
Generally the electric field for a point is in an insulator(rubber) is mathematically represented as
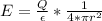
Where
 is the permittivity of rubber
is the permittivity of rubber
=>
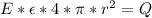
=>
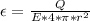
substituting values