Answer:
Arkansas students have higher debt than the national average, $27,200.
Step-by-step explanation:
The provided data is:
S = {24040, 19153, 26762, 31923, 31533, 34207, 14623, 24370, 31016}
In this case we need to test whether Arkansas students have higher debt than the national average at alpha equal to 0.10.
The hypothesis can be defined as follows:
H₀: Arkansas students does not have higher debt than the national average, i.e. μ ≤ $27,200.
Hₐ: Arkansas students have higher debt than the national average, i.e. μ > $27,200.
Compute the sample mean:
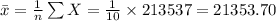
As the population standard deviation is provided, we will use a z-test for single mean.
Compute the test statistic value as follows:
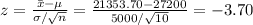
The test statistic value is -3.70.
Decision rule:
If the p-value of the test is less than the significance level then the null hypothesis will be rejected.
Compute the p-value for the test as follows:
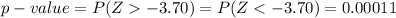
*Use a z-table for the probability.
The p-value of the test is 0.00011.
p-value = 0.00011 < α = 0.10
The null hypothesis will be rejected.
Thus, it can be concluded that Arkansas students have higher debt than the national average, $27,200.