Question

Find the surface area of each prism. Round to the nearest tenth if necessary while doing your calculations as well as in your final answer. 360 units2 586 units2 456 units2 552 units2

Answer 1

correct answer is 456 sq units.

Explanation:

Let us have a look at the formula for Surface Area of a prism:

`A =p * h+2 * B`

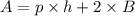
Where p is the perimeter of base

h is the height of prism

and B is the base area of prism.

Given that:

h = 7.5 units

Hypotenuse of prism's base = 20 units

One of the Other sides = 12 units

Pythagorean theorem can be used to find the 3rd side of right angled base.

Square of hypotenuse = Sum of squares of other two sides

`20^2=12^2+side^2\\\Rightarrow 400=144+side^2\\\Rightarrow side =√(256)\\\Rightarrow side =16\ units`

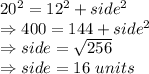
Area of base = area of right angled triangle:

`B = (1)/(2) * \text{Base Length} * \text{Perpendicular Length}\\\Rightarrow B = (1)/(2) * 16* 12 = 96\ sq\ units`

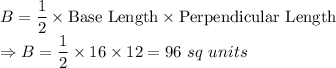
Perimeter
`*` height = (12+20+16)
`*` 7.5 = (48)
`*` 7.5 = 360 sq units

Now putting the values in formula:

Surface area, A = 360+96 = 456 sq units

So, correct answer is 456 sq units.