Question

Integrate the following: ∫84
`dx`

A. 42x
B. 84x
C. 84x + C
D. 42x + C

Answer 1

If a is a constant then it's inetgration is

`\boxed{\sf \displaystyel\int adx=ax+C}`

• Here 84 is constant

`\\ \rm\Rrightarrow \displaystyle\int 84dx`

`\\ \rm\Rrightarrow 84x+C`

Option C

Answer 2

Choice C.
`84\, x + C`.

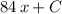Explanation:

Consider the power rule for integration. Let
`n` be a real number that is not equal to
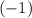`(-1)`. The power rule for integration states that:

`\displaystyle \int x^(n)\, d x = (1)/(n + 1)\, x^(n+ 1) + C`,

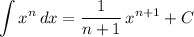How could this rule apply to this question, since there's apparently no
`x` (or its powers) in the integrand? Keep in mind that
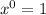`x^(0) = 1` for all real (and particularly non-zero) values of
`x`. In other words, the integrand
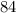`84` is equal to
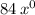`84\, x^0`. The integral becomes:

`\displaystyle \int 84\, x^(0)\, dx`.

The constant can be moved outside the integral sign. Therefore:

`\displaystyle \int 84\, x^(0)\, dx= 84 \int x^(0)\, dx`.

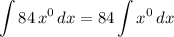Now that resembles the power rule. In particular,
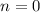`n = 0`, such that
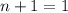`n + 1 = 1`. By the power rule:

`\begin{aligned}84 \int x^(0)\, dx = 84\, \left((1)/(1)\, x^(1) + C\right) = 84\, x + 84\, C\end{aligned}`.

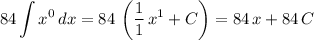The non-zero constant in front of
`C` can be ignored (where
`C` represents the constant of integration.) Therefore:

`\displaystyle \int 84\, dx = 84\, x + C`.