Question

Integrate the following: ∫
`5x^4dx`

A.
`x^5+C`
B.
`x^5`
C.
`5x^5+C`
D.
`5x^5`

Answer 1

Answer A

Explanation:

Use the property of integrals. You now have
`5 x\int\limits\,x^(4)dx` where the first x next to the 5 stands for multiplication. Let's evaluate it. We get
`5 ((x^(5) )/(5))`. From here, we can simplify this into
`x^(5)`. Add the constant of integration, which will give you the answer of
`x^(5) + C`.

Answer 2

A.
`x^5+C`

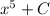
Explanation:

This is a great question! The first thing we want to do here is to take the constant out of the expression, in this case 5. Doing so we would receive the following expression -

`5\cdot \int \:x^4dx`

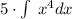
We can then apply the power rule "
`\int x^adx=(x^(a+1))/(a+1)` ", where a = exponent ( in this case 4 ),

`5\cdot (x^(4+1))/(4+1)`

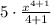
From now onward just simplify the expression as one would normally, and afterward add a constant ( C ) to the solution -

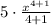`5\cdot (x^(4+1))/(4+1)\\` - Add the exponents,

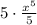`5\cdot (x^(5))/(5)` - 5 & 5 cancel each other out,

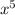`x^5` - And now adding the constant we see that our solution is option a!