Answer:
(Choice C) C Infinitely many solutions.
Explanation:
First of all, let us learn about solutions of linear equations in one variable.
The linear equations in one variable usually have one solution.
For example:
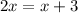
When we solve this:
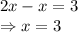
One solution is

But there can be situations when there are
1. No solutions:
For example:
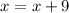
It means that value x is equal to value of x+9 which can never be true.
Truth is the term on Right Hand Side is always 9 greater than the value of Left Hand Side.
Such situations are called Contradictions.
Here, no solution exists.
2. Infinitely many solutions:
For example:
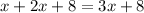
The Right hand Side is just the simplification of the LHS.
And LHS is always equal to RHS no matter what is the value of variable
 .
.
It means there are infinitely many solutions for this equation.
-----------------------------------------------------
Now, let us have a look at the given equation in the question:
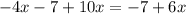
Taking LHS:

Taking the terms with
 on one side:
on one side:
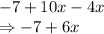
which is equal to Right Hand Side.
Hence, as we discussed in case 2 above.
For every value of
 the equation holds true.
the equation holds true.
 There exists infinitely many solutions to the given equation.
There exists infinitely many solutions to the given equation.
Correct answer is:
(Choice C) C Infinitely many solutions