Question

asked Dec 24, 2023 226k views

2 Answers

Jayprakash Singh · Answer 1 · 2023-12-26T14:02:53+0000

- - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - -

$\blue{\textsf{\textbf{\underline{\underline{Question:-}}}}$

What are the next two terms of the following sequence?

$\blue{\textsf{\textbf{\underline{\underline{Answer:-}}}}$

The next two terms are -32 and 64.

$\blue{\textsf{\textbf{\underline{\underline{How to Solve:-}}}}$

First of all, let's identify what kind of sequence this is.

The two main types of sequences are:

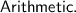$\textsf{Arithmetic.}$ This is where we add/subtract the same number to get to the following term.
$\textsf{Geometric.}$ This is where we multiply/divide by the same number to get to the next term.

In the given sequence, we use multiplication, so we're dealing with a
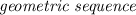$\textit{geometric sequence}$ .

In order to get to the next term, we multiply by -2.

So in order to find the next two terms of the sequence, we take the previous term and multiply it by -2 as follows:

16•(-2)=-32

We've found the 5th term of the geometic sequence.

Now let's find the 6th:

-32•(-2)=64 (the product of a negative and a negative results in a positive)

The next two terms in the sequence are -32 and 64.

- - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - -

Salisha · Answer 2 · 2023-12-28T10:52:15+0000

Answer:

-32 , 64

Explanation:

Geometric sequence

This is a geometric sequaence and each term is obtatained by multiplying the previous number with the common ratio 'r'

$\sf \boxed{r = (second \ term )/(first \ term)}$

$\sf r =(4)/((-2))$

r = -2

The next two terms ,

Fifth term = 16 *(-2) = -32

Sixth term = (-32) * (-2) = 64