Question

Two spherical objects at the same altitude move with identical velocities and experience the same drag force at a time t. If Object 1 has twice (2x) the diameter of Object 2, which object has the larger drag coefficient? Explain your answer using the drag equation.

Answer 1

The object with the twice the area of the other object, will have the larger drag coefficient.

Step-by-step explanation:

The equation for drag force is given as

`F_(D) = (1)/(2)pu^(2) C_(D) A`

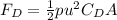
where
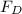`F_(D)` IS the drag force on the object

p = density of the fluid through which the object moves

u = relative velocity of the object through the fluid

p = density of the fluid

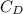`C_(D)` = coefficient of drag

A = area of the object

Note that
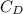`C_(D)` is a dimensionless coefficient related to the object's geometry and taking into account both skin friction and form drag. The most interesting things is that it is dependent on the linear dimension, which means that it will vary directly with the change in diameter of the fluid

The above equation can also be broken down as

`F_(D)` ∝
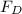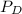`P_(D)` A

where
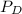`P_(D)` is the pressure exerted by the fluid on the area A

Also note that
`P_(D)` =
`(1)/(2)pu^(2)`

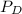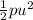
which also clarifies that the drag force is approximately proportional to the abject's area.

In this case, the object with the twice the area of the other object, will have the larger drag coefficient.