Question

Use Bayes' theorem to find the indicated probability 5.8% of a population is infected with a certain disease. There is a test for the disease, however the test is not completely accurate. 93.9% of those who have the disease test positive. However 4.1% of those who do not have the disease also test positive (false positives). A person is randomly selected and tested for the disease. What is the probability that the person has the disease given that the test result is positive?

a. 0.905
b. 0.585
c. 0.038
d. 0.475

Answer 1

b. 0.585

Explanation:

According to Bayes' theorem:

`P(A|B)=(P(B|A)*P(A))/(P(B))`

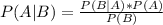
Let A = Person is infected, and B = Person tested positive. Then:

P(B|A) = 93.9%

P(A) = 5.8%

P(B) = P(infected and positive) + P(not infected and positive)

`P(B) = 0.058*0.939+(1-0.058)*0.041\\P(B)=0.09308`

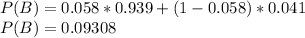
Therefore, the probability that a person has the disease given that the test result is positive, P(A|B), is:

`P(A|B)=(0.939*0.058)/(0.09308)\\P(A|B)=0.585`

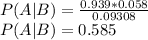
The probability is 0.585.