Answer:
C. $1030.34
Step-by-step explanation:
Given:
Principal = $1,000
Interest = 3%
Number of Times = Quarterly
Time = 1 year
Interest Type = Compound Interest
Required
Amount at the end of the year
To solve this, I'll make use of Amount formula which is;

Using compound interest notations, the given parameters are:
P = $1,000
r = 3%
t = 1
Quarterly means 4 times a year;
So, n = 4
Solving for Amount (A), the formula becomes
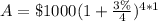
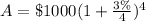
Convert percent to decimal
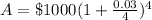
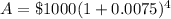
Solve the expression in the bracket

Solve the exponent
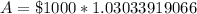
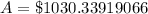
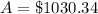 (Approximated)
(Approximated)
Hence, the amount at the end of the year is approximately $1030.34