Answer:
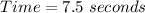
Explanation:
Given
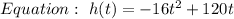
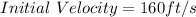
Required:
Determine the time taken to return to the ground
From the equation given; height (h) is a function of time (t)
When the rocket returns to the ground level, h(t) = 0
Substitute 0 for h(t) in the given equation
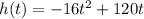
becomes
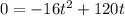
Solve for t in the above equation

Factorize the above expression
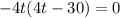
Split the expression to 2
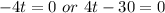
Solving the first expression
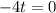
Divide both sides by -4

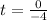
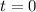
Solving the second expression
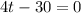
Add 30 to both sides
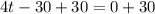

Divide both sides by 4
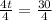
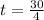
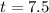
Hence, the values of t are:
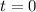 and
and
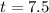
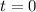 shows the time before the launching the rocket
shows the time before the launching the rocket
while
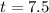 shows the time after the rocket returns to the floor
shows the time after the rocket returns to the floor