Question

An ideal gas initially at 300 K undergoes an isobaric expansion at 2.50 kPa. If the volume increases from 1.00 m3 to 3.00 m3 and 12.5 kJ is transferred to the gas by heat, what are a. the change in its internal energy and b. its final temperature?

Answer 1

a)
`\Delta U = 7.5 kJ`

b)
`T_(f) = 900 K`

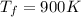
Step-by-step explanation:

a) To find the change in its internal energy (U) we need to use the following equation:

`\Delta U = W + Q`

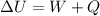
Where:

W: is the work done on the system

Q: is the energy transferred into the system by heat = 12.5 kJ

Since we have an isobaric expansion, the work is:

`W = - P\Delta V = - P(V_(f) - V_(i))`

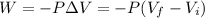
Where:

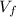`V_(f)`: is the final volume = 3.00 m³

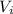`V_(i)`: is the initial volume = 1.00 m³

P: is the pressure = 2.50 kPa

`W = -P(V_(f) - V_(i)) = -2.5 \cdot 10^(3) Pa(3.00 m^(3) - 1.00 m^(3)) = -5.00 \cdot 10^(3) J`

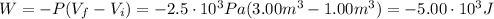
Now, we can find the change in its internal energy:

`\Delta U = W + Q = -5.00 \cdot 10^(3) J + 12.5 \cdot 10^(3) J = 7.5 \cdot 10^(3) J`

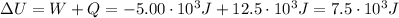
b) The final temperature can be found as follows:

`(V_(i))/(V_(f)) = (T_(i))/(T_(f))`

`T_(f) = (T_(i)*V_(f))/(V_(i)) = (300 K*3.00 m^(3))/(1.00 m^(3)) = 900 K`

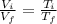
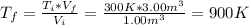
Hence, the final temperature is 900 K.

I hope it helps you!