Question

a sample of 23.2 grams of nitrogen gas is reacted with 23.2 G of hydrogen gas to produce ammonia. using the balanced equation below predict which of the reactants is the limiting reactant ​

Answer 1

Nitrogen gas is the limiting reactant.

To determine the limiting reactant, we first need to write the balanced chemical equation for the reaction between nitrogen gas and hydrogen gas to produce ammonia. The balanced equation is:

`\[ N_2(g) + 3H_2(g) \rightarrow 2NH_3(g) \]`

Now, let's use the given masses to calculate the moles of each reactant:

Moles of
`\(N_2\)`:

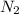`\[ \text{Moles} = \frac{\text{Mass}}{\text{Molar Mass}} = \frac{23.2 \, \text{g}}{28.02 \, \text{g/mol}} \approx 0.826 \, \text{mol} \]`

Moles of \(H_2\):

`\[ \text{Moles} = \frac{\text{Mass}}{\text{Molar Mass}} = \frac{23.2 \, \text{g}}{2.016 \, \text{g/mol}} \approx 11.5 \, \text{mol} \]`

Now, using the coefficients from the balanced equation, we can determine the stoichiometric ratio of moles:

`\[ \frac{\text{Moles of } N_2}{1} = \frac{\text{Moles of } H_2}{3} \]`

Since the actual ratio is
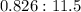`\(0.826 : 11.5\)`, which is less than
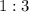`\(1 : 3\)`, nitrogen gas
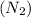`(\(N_2\))` is the limiting reactant.

Answer 2

Hydrogen

Step-by-step explanation:

First thing's first, we have to write out the balanced chemical equation for the reaction.

This is given as;

N2 + 3H2 --> 2NH3

From the stoichiometrey of the reaction;

Molar mass of N = 14

Molar mass of H = 1

(1 * (14 * 2)) = 28g of N2 reacts with (3 * (1 * 2)) = 6g of H2

This means that if there are equal mass of both Nitrogen and Hydrogen. We would run out of Hydrogen first. This means Hydrogen is our limiting reactant as it determines the amount of products that can be formed.