Question

The sum of the first two terms of an infinite GP is 6 and each term is 5 times the sum of the succeeding termsthen the second term GP is

Answer 1

`(6)/(7)`.

Explanation:

It is given that the sum of first two terms of an infinite GP is 6.

nth term of a GP is

`a_n=ar^(n-1)`

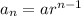
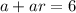`a+ar=6` ...(1)

Each term is 5 times the sum of the succeeding terms.

`a_n=5(a_(n+1)+a_(n+2)+...+\infty)`

`ar^(n-1)=5(ar^n+ar^(n+1)+...+\infty)`

`ar^(n-1)=5ar^n(1+r+r^2+...+\infty)`

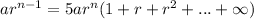
Divide both sides by a.

`r^(n-1)=5r^n((1)/(1-r))`
`[\text{Sum of infinite GP}=(a)/(1-r)]`

`(r^n)/(r)=(5r^n)/(1-r)`

`(1)/(r)=(5)/(1-r)`

`1-r=5r`

`1=6r`

`(1)/(6)=r`

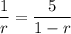
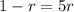
The common ratio is 1/6.

Put r=1/6 in (1).

`a+a((1)/(6))=6`

`6a+a=36`

`7a=36`

`a=(36)/(7)`

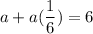
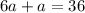
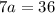
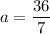
Second term
`ar=(36)/(7)* (1)/(6)=(6)/(7)`

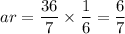
Therefore, the second term is
`(6)/(7)`.