Question

A(7-1), B(6, 4) and C(5, 5) are three

points in a plane.
1). Find the equations of the
perpendicular bisectors of AB and AC
(ü) Determine the point of
intersection of the perpendicular
bisectors in (i).
(WAEC)​

Answer 1

The answer is below

Explanation:

The equation of the line passing through two points is given by:

`y-y_1=(y_2-y_1)/(x_2-x_1)(x-x_1)\\`

The equation of line AB is:

`y-y_1=(y_2-y_1)/(x_2-x_1)(x-x_1)\\\\y-(-1)=(4-(-1))/(6-7)(x-7)\\\\y+1=-5(x-7)\\y+1=-5x+35\\y=-5x+34`

The midpoint of two lines is given as:

`x=(x_1+x_2)/(2), y=(y_1+y_2)/(2)\\midpoint\ of\ AB \ is:\\x=(7+6)/(2)=6.5, y=(-1+4)/(2)=1.5\\ = (6.5,1.5)`

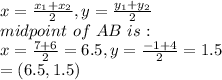
The equation of line AC is:

`y-y_1=(y_2-y_1)/(x_2-x_1)(x-x_1)\\\\y-(-1)=(5-(-1))/(5-7)(x-7)\\\\y+1=-3(x-7)\\y+1=-3x+21\\y=-3x+20`

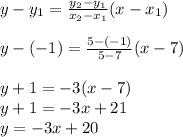
The midpoint of two lines is given as:

`x=(x_1+x_2)/(2), y=(y_1+y_2)/(2)\\midpoint\ of\ AB \ is:\\x=(7+5)/(2)=6, y=(-1+5)/(2)=2\\ = (6,2)`

The product of the slope of a perpendicular bisector of a line and the slope of the line is -1. That is m1m2 = -1

The slope of the perpendicular bisector of AB is:

m(-5)=-1

m=1/5

The equation of the perpendicular bisector of AB passing through (6.5,1.5) is:

`y-y_1=m(x-x_1)\\y-1.5=(1)/(5)(x-6.5)\\y=(1)/(5) x-8.25`

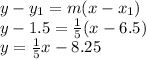
The slope of the perpendicular bisector of AB is:

m(-3)=-1

m=1/3

The equation of the perpendicular bisector of AB passing through (6,2) is:

`y-y_1=m(x-x_1)\\y-2=(1)/(3)(x-7)\\y=(1)/(3) x-4.33`

2) The point of intersection is gotten by solving y = 1/5 x -8.25 and y = 1/3 x-4.33 simultaneously.

Subtracting the two equations from each other gives:

0= -0.133x - 3.92

-0.133x = 3.92

x = -29.5

Put x = -29.5 in y = 1/5 x -8.25 i.e:

y = 1/5 (29.5) -8.25

y = -14.16

The point of intersection is (-29.5, -14.16)