Question

X – 6x² + 3x - 18=0

What is the only value of x that is a real solution to the
equation shown above?

Answer 1

x=6

Explanation:

The equation ...

`x^3-6x^2+3x-18=0`

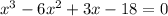
can be factored by grouping to get a linear factor and a quadratic factor. The linear factor always has a real zero. This quadratic factor does not.

`=(x^3-6x^2)+(3x-18)=x^2(x-6)+3(x-6)\\\\=(x-6)(x^2+3)=0`

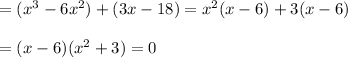
The one real solution is x=6, the value of x that makes (x-6) = 0.