Answer: b.
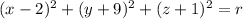
Explanation:
The general equation of a sphere is :
 , where (a, b, c) represents the center of the sphere, R represents the radius, and x, y, and z are the coordinates of the points on the surface of the sphere.
, where (a, b, c) represents the center of the sphere, R represents the radius, and x, y, and z are the coordinates of the points on the surface of the sphere.
Given, Center of the sphere = (2,-9,-1)
Radius = √r
Then, the equation of the sphere would be
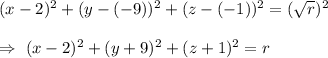
Hence, the correct option is b.
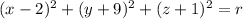 .
.